
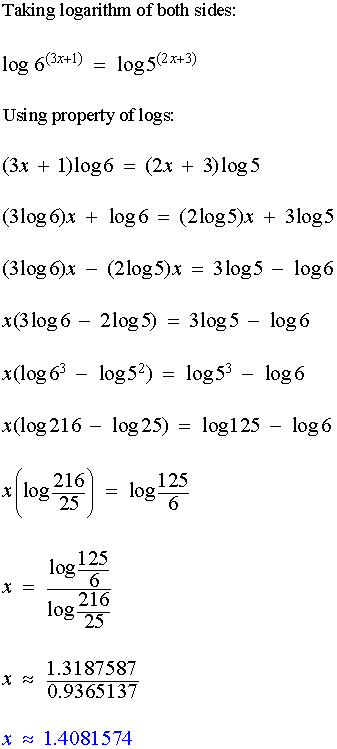
Suppose an investigator had developed a scoring system that enabled her to predict an individual's body mass index (BMI) based on information about what they ate and how much. To provide an intuitive understanding of how multiple linear regression does this, consider the following hypothetical example. This is a very useful procedure for identifying and adjusting for confounding. However, if there were other factors (independent variables) that influenced body weight besides height (e.g., age, calorie intake, and exercise level), we might expect that the points for individual subjects would be more loosely scattered around the line, since we are only taking height into account.
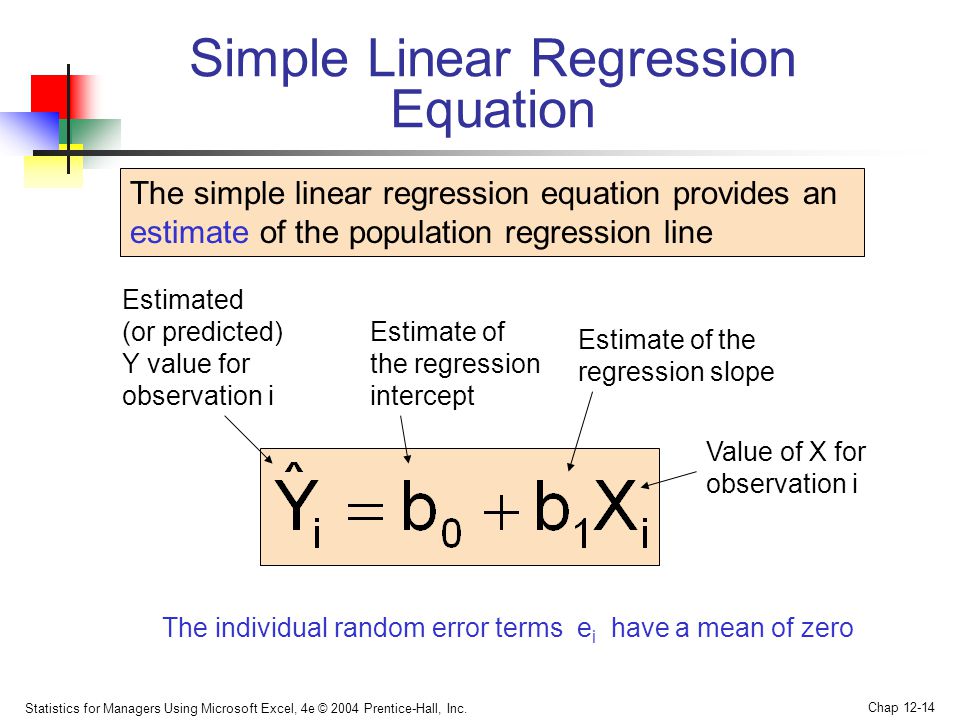
If height were the only determinant of body weight, we would expect that the points for individual subjects would lie close to the line. In this simple linear regression, we are examining the impact of one independent variable on the outcome. In this example, if an individual was 70 inches tall, we would predict his weight to be: We could use the equation to predict weight if we knew an individual's height. We could also describe this relationship with the equation for a line, Y = a + b(x), where 'a' is the Y-intercept and 'b' is the slope of the line. If we were to plot height (the independent or 'predictor' variable) as a function of body weight (the dependent or 'outcome' variable), we might see a very linear relationship, as illustrated below. For example, suppose that height was the only determinant of body weight.
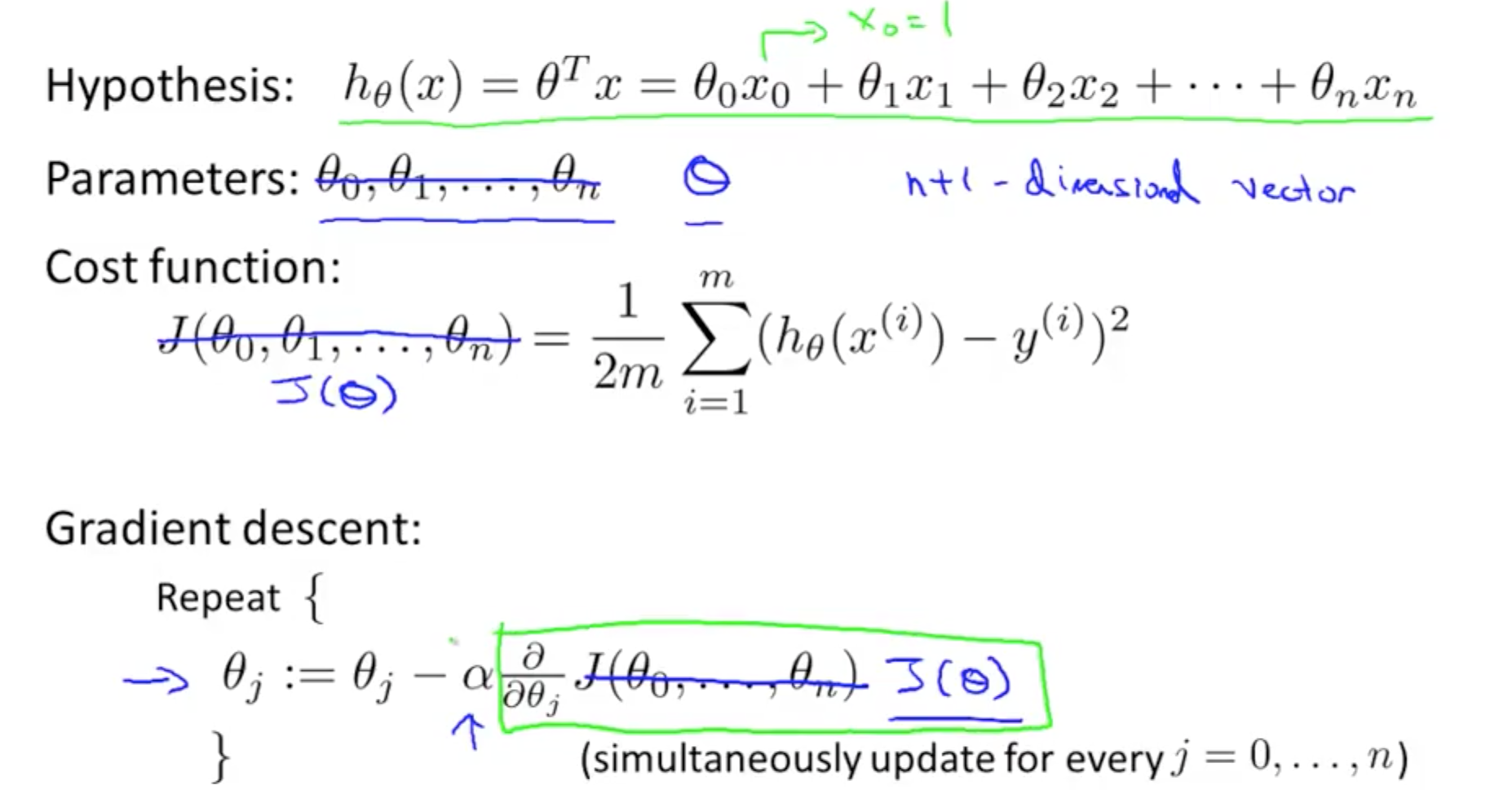
Regression analysis makes use of mathematical models to describe relationships. LaMorte, MD, PhD, MPH, Professor of Epidemiologyīoston University School of Public Health Python Dictionaries Access Items Change Items Add Items Remove Items Loop Dictionaries Copy Dictionaries Nested Dictionaries Dictionary Methods Dictionary Exercise Python If.Else Python While Loops Python For Loops Python Functions Python Lambda Python Arrays Python Classes/Objects Python Inheritance Python Iterators Python Scope Python Modules Python Dates Python Math Python JSON Python RegEx Python PIP Python Try.Lisa Sullivan, Professor of Biostatistics


 0 kommentar(er)
0 kommentar(er)
